Comparative Analysis on the Effect of Crack Location and Orientation on Crack Growth in Boiler Tube

Majeed ur Rahman; Babar Saeed

Authors

Majeed ur Rahman Institute of Avionics and Aerospace, Air University, Islamabad, Pakistan
Babar Saeed Institute of Avionics and Aerospace, Air University, Islamabad, Pakistan

Keywords:

Tubes, Fracture Mechanics, Weight Function, Stress Intensity Factor, Crack Growth, ANSYS SMART, Simpson’s Integration

Abstract

Introduction/Importance of Study:

Safety is the paramount concern in the operations and inspections of pressure vessels, such as water tube boilers. Defects in the boiler tubes can lead to the development of cracks.

Novelty Statement:

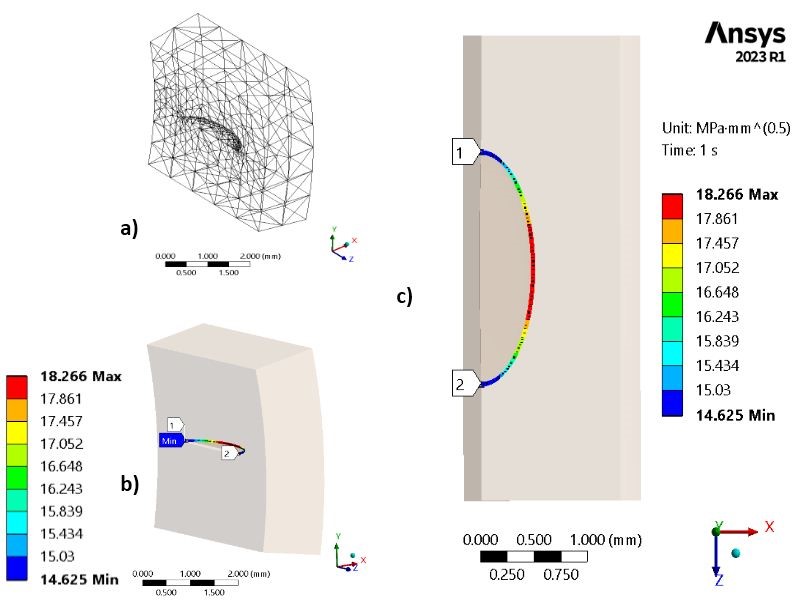
The investigation focuses on the effect of crack location and orientation on crack growth under cyclic loading which has been analyzed computationally using Separate Morphing and Re-meshing Technology (SMART) in ANSYS. The effect of location on crack growth is primarily focused which is theoretically investigated as well using Simpson’s Integration of Paris’s Law.

Materials and Method:

The tube in focus is a component of a D-type water-tube industrial boiler, crafted from low-carbon steel (SA 178 A). For the effect of location, semi-elliptical cracks on inner and outer tube surfaces have been studied both theoretically and computationally.

Results and Discussion:

Theoretical investigation revealed that cracks on the inner tube surface exhibit a 30.28% higher accumulative growth rate compared to the outer surface, attributed to hoop stress distribution. For investigating the effect of orientation elliptical embedded cracks at certain orientations have been examined computationally and the critical plane orientation for crack growth is identified as perpendicular to the hoop stress.

Concluding Remarks:

In conclusion, the study underscores that cracks grow faster when located at the inner surface and oriented perpendicular to the hoop stress.

References

“Experimental investigation of boiler blast load on building structures.” Accessed: Mar. 18, 2024. [Online]. Available: http://lib.buet.ac.bd:8080/xmlui/bitstream/handle/123456789/6243/Full Thesis.pdf?sequence=1&isAllowed=y

A. R. Paul, F. Alam, A. Jain, and M. S. Ali, “Boiler Safety in South Asia,” J. Inst. Eng. Ser. C, vol. 101, no. 5, pp. 761–769, Oct. 2020, doi: 10.1007/S40032-020-00597-0/METRICS.

C. Bierl, “Boiler and Pressure Vessel Safety, Hazards in the Workplace!” OnePetro, Jun. 19, 2017. Accessed: Mar. 18, 2024. [Online]. Available: https://dx.doi.org/

A. Toudehdehghan and T. W. Hong, “A critical review and analysis of pressure vessel structures,” IOP Conf. Ser. Mater. Sci. Eng., vol. 469, no. 1, p. 012009, Jan. 2019, doi: 10.1088/1757-899X/469/1/012009.

K. R. Golwalkar and R. Kumar, “Pressure Vessels,” Pract. Guidel. Chem. Ind., pp. 55–80, 2022, doi: 10.1007/978-3-030-96581-5_4.

D. Ghosh, H. Roy, A. Saha, and C. Subramanian, “Failure Analysis of Boiler Water Wall Tube: A Case Study from Thermal Power Plant,” J. Fail. Anal. Prev., vol. 22, no. 1, pp. 203–208, Feb. 2022, doi: 10.1007/S11668-021-01271-Y/METRICS.

E. Febriyanti, A. Suhadi, and L. N. Sari, “FATIGUE AND CORROSION PHENOMENON ON FAILURE OF WATER WALL TUBE BOILER,” Maj. Ilm. Pengkaj. Ind. J. Ind. Res. Innov., vol. 14, no. 1, pp. 29–38, Sep. 2020, doi: 10.29122/MIPI.V14I1.3565.

F. Bjørheim, S. C. Siriwardane, and D. Pavlou, “A review of fatigue damage detection and measurement techniques,” Int. J. Fatigue, vol. 154, p. 106556, Jan. 2022, doi: 10.1016/J.IJFATIGUE.2021.106556.

“API 579-1 FFS Features in INSPECT - Codeware.” Accessed: Mar. 18, 2024. [Online]. Available: https://www.codeware.com/products/inspect/api-579-1/?gad_source=1

R. Usamentiaga, D. G. Lema, O. D. Pedrayes, and D. F. Garcia, “Automated surface defect detection in metals: A comparative review of object detection and semantic segmentation using deep learning,” Conf. Rec. - IAS Annu. Meet. (IEEE Ind. Appl. Soc., vol. 2021-October, 2021, doi: 10.1109/IAS48185.2021.9677231.

R. Sui, Y. Zhao, B. Ge, and W. Wang, “Failure analysis of leakage at tube-to-tubesheet joints of a waste heat boiler,” Eng. Fail. Anal., vol. 129, p. 105639, Nov. 2021, doi: 10.1016/J.ENGFAILANAL.2021.105639.

E. S. Kim, “Fracture analysis of tube boiler for physical explosion accident,” Forensic Sci. Int., vol. 278, pp. e1–e7, Sep. 2017, doi: 10.1016/J.FORSCIINT.2017.07.036.

N. H. Lee, S. Kim, B. H. Choe, K. B. Yoon, and D. il Kwon, “Failure analysis of a boiler tube in USC coal power plant,” Eng. Fail. Anal., vol. 16, no. 7, pp. 2031–2035, Oct. 2009, doi: 10.1016/J.ENGFAILANAL.2008.12.006.

N. V. Challenger, R. Phaal, and S. J. Garwood, “Fracture mechanics assessment of industrial pressure vessel failures,” Int. J. Press. Vessel. Pip., vol. 61, no. 2–3, pp. 433–456, Jan. 1995, doi: 10.1016/0308-0161(94)00120-8.

A. Malik, A. Meroufel, and S. Al-Fozan, “Boiler Tubes Failures: A Compendium of Case Studies,” J. Fail. Anal. Prev., vol. 15, no. 2, pp. 246–250, Apr. 2015, doi: 10.1007/S11668-015-9923-X/METRICS.

“Materials Science and Engineering”, [Online]. Available: https://www.wiley.com/en-gb/Callister%27s+Materials+Science+and+Engineering%2C+Global+Edition%2C+10th+Edition-p-9781119453918

T. L. Anderson, “FRACTURE MECHANICS: Fundamentals and Applications, Fourth Edition,” Fract. Mech. Fundam. Appl. Fourth Ed., pp. 1–661, Jan. 2017, doi: 10.1201/9781315370293/FRACTURE-MECHANICS-TED-ANDERSON.

N. Pugno, M. Ciavarella, P. Cornetti, and A. Carpinteri, “A generalized Paris’ law for fatigue crack growth,” J. Mech. Phys. Solids, vol. 54, no. 7, pp. 1333–1349, Jul. 2006, doi: 10.1016/J.JMPS.2006.01.007.

D. Rozumek and E. MacHa, “A survey of failure criteria and parameters in mixed-mode fatigue crack growth,” Mater. Sci., vol. 45, no. 2, pp. 190–210, Mar. 2009, doi: 10.1007/S11003-009-9179-2/METRICS.

H. Yuan, W. Yang, L. Zhang, and T. Hong, “Model Development of Stress Intensity Factor on 7057T6 Aluminum Alloy Using Extended Finite Element Method,” Coatings 2023, Vol. 13, Page 581, vol. 13, no. 3, p. 581, Mar. 2023, doi: 10.3390/COATINGS13030581.

“AFGROW (Air Force Growth) Fracture Mechanics and Fatigue Crack Growth Analysis Software - Home Page.” Accessed: Mar. 18, 2024. [Online]. Available: https://www.afgrow.net/

Y. A. Fageehi and A. M. Alshoaibi, “Nonplanar Crack Growth Simulation of Multiple Cracks Using Finite Element Method,” Adv. Mater. Sci. Eng., vol. 2020, 2020, doi: 10.1155/2020/8379695.

D. D’Angela and M. Ercolino, “Fatigue crack growth in metallic components: numerical modeling and analytical solution,” Authorea Prepr., Jul. 2020, doi: 10.22541/AU.159493143.38339244.

T. T. Htut et al., “Fatigue fracture investigation of a tube-to-tubesheet welded joint,” Eng. Struct., vol. 283, p. 115908, May 2023, doi: 10.1016/J.ENGSTRUCT.2023.115908.

J. Niu and M. S. Wu, “Analysis of asymmetric kinked cracks of arbitrary size, location and orientation - Part I. Remote compression,” Int. J. Fract., vol. 89, no. 1, pp. 19–57, 1998, doi: 10.1023/A:1007428827074/METRICS.

J. Niu and M. S. Wu, “Analysis of asymmetric kinked cracks of arbitrary size, location and orientation - Part II. Remote tension,” Int. J. Fract., vol. 89, no. 1, pp. 59–84, 1998, doi: 10.1023/A:1007476710235/METRICS.

G. de C. Coêlho, A. A. Silva, M. A. Santos, A. G. B. Lima, and N. C. Santos, “Stress Intensity Factor of Semielliptical Surface Crack in Internally Pressurized Hollow Cylinder—A Comparison between BS 7910 and API 579/ASME FFS-1 Solutions,” Mater. 2019, Vol. 12, Page 1042, vol. 12, no. 7, p. 1042, Mar. 2019, doi: 10.3390/MA12071042.

S. A. Ligoria, G. M. S. Knight, and D. S. Ramachandra Murthy, “Three-dimensional Finite Element Analysis of a Semi-Elliptical Circumferential Surface Crack in a Carbon Steel Pipe Subjected to a Bending Moment,” http://dx.doi.org/10.1243/030932405X16052, vol. 40, no. 6, pp. 525–533, Aug. 2005, doi: 10.1243/030932405X16052.

“Metallography and Microstructures,” Metallogr. Microstruct., Dec. 2004, doi: 10.31399/ASM.HB.V09.9781627081771.

C. D. Wallbrink, D. Peng, and R. Jones, “Assessment of partly circumferential cracks in pipes,” Int. J. Fract., vol. 133, no. 2, pp. 167–181, May 2005, doi: 10.1007/S10704-005-0628-0/METRICS.

A. Zareei and S. M. Nabavi, “Weight function for circumferential semi-elliptical cracks in cylinders due to residual stress fields induced by welding,” Arch. Appl. Mech., vol. 86, no. 7, pp. 1219–1230, Jul. 2016, doi: 10.1007/S00419-015-1087-3/METRICS.

S. Melin, “Which is the most unfavourable crack orientation?,” Int. J. Fract., vol. 51, no. 3, pp. 255–263, Oct. 1991, doi: 10.1007/BF00045811/METRICS.

D. L. McDowell, “Basic issues in the mechanics of high cycle metal fatigue,” Int. J. Fract., vol. 80, no. 2, pp. 103–145, Apr. 1989, doi: 10.1007/BF00012666/METRICS.

A. Karolczuk and E. Macha, “A review of critical plane orientations in multiaxial fatigue failure criteria of metallic materials,” Int. J. Fract., vol. 134, no. 3–4, pp. 267–304, Aug. 2005, doi: 10.1007/S10704-005-1088-2/METRICS.

A. Moftakhar, A. Buczynski, and G. Glinka, “Calculation of elasto-plastic strains and stresses in notches under multiaxial loading,” Int. J. Fract., vol. 70, no. 4, pp. 357–373, Dec. 1994, doi: 10.1007/BF00032453/METRICS.

M. Kamaya and T. Kitamura, “Stress intensity factors of microstructurally small crack,” Int. J. Fract., vol. 124, no. 3–4, pp. 201–213, 2003, doi: 10.1023/B:FRAC.0000018238.41283.A4/METRICS.

“Simulate of edge and an internal crack problem and estimation of stress intensity factor through finite element method.” Accessed: Mar. 18, 2024. [Online]. Available: https://www.techno-press.org/content/?page=article&journal=anr&volume=12&num=4&ordernum=6

O. Elmhaia, Y. Belaasilia, O. Askour, B. Braikat, and N. Damil, “An efficient mesh-free approach for the determination of stresses intensity factors,” Eng. Anal. Bound. Elem., vol. 133, pp. 49–60, Dec. 2021, doi: 10.1016/J.ENGANABOUND.2021.08.001.

P. K. Pati, S. K. Shrivastava, and S. Basu, “Numerical analysis of crack initiation and growth in cylindrical geometries with an axial flaw,” Int. J. Fract., vol. 148, no. 4, pp. 291–301, Dec. 2007, doi: 10.1007/S10704-008-9202-X/METRICS.

C. D. M. O. Ranaraja, J. W. Devasurendra, M. I. P. Maduwantha, G. A. L. Madhuwantha, and R. Y. D. Hansa, “Optimization of an Industrial Boiler Operation,” J. Res. Technol. Eng., vol. 1, 2020.

A. Chaudouet, F. Osweiller, P. Hanmore, and G. G. Karcher, “Perspectives of the Pressure Equipment Directive with Respect to ASME BPVC,” Companion Guid. to ASME Boil. Press. Vessel Code, Vol. 3, Third Ed., pp. 129–157, Nov. 2009, doi: 10.1115/1.802717.CH47.

I. Mazínová and P. Florian, “Materials selection in mechanical design,” Lect. Notes Mech. Eng., vol. 16, pp. 145–153, 2014, doi: 10.1007/978-3-319-05203-8_21.

ASME (American Society of Mechanical Engineers), “Asme Bpvc,” Asme Boil. Press. Vessel Code, 2015.

“Shigley’s Mechanical Engineering Design - Richard Budynas, Keith Nisbett - Google Books.” Accessed: Mar. 18, 2024. [Online]. Available: https://books.google.com.pk/books/about/Shigley_s_Mechanical_Engineering_Design.html?id=B7wivgAACAAJ&redir_esc=y

D. Annaratone, “Pressure vessel design,” Press. Vessel Des., pp. 1–443, 2007, doi: 10.1007/978-3-540-49144-6/COVER.

“Polar contour plot - File Exchange - MATLAB Central.” Accessed: Mar. 18, 2024. [Online]. Available: https://www.mathworks.com/matlabcentral/fileexchange/14826-polar-contour-plot

X. R. Wu and W. Xu, “Weight Function Methods in Fracture Mechanics: Theory and Applications,” Weight Funct. Methods Fract. Mech. Theory Appl., pp. 1–654, Jan. 2022, doi: 10.1007/978-981-16-8961-1/COVER.

“WEIGHT FUNCTION METHODS AND ASSESSMENT FOR AN EDGE CRACK IN A SEMI-INFINITE PLATE 1,” vol. 49, no. 4, pp. 848–857, 2017, [Online]. Available: https://pubs-en.cstam.org.cn/article/doi/10.6052/0459-1879-17-024

“Universal features of weight functions for cracks in mode I - ScienceDirect.” Accessed: Mar. 18, 2024. [Online]. Available: https://www.sciencedirect.com/science/article/abs/pii/0013794491901773?via%3Dihub

U. A. Campos and D. E. Hall, “Simplified Lamé’s equations to determine contact pressure and hoop stress in thin-walled press-fits,” Thin-Walled Struct., vol. 138, pp. 199–207, May 2019, doi: 10.1016/J.TWS.2019.02.008.

X. J. Zheng, A. Kiciak, and G. Glinka, “Weight functions and stress intensity factors for internal surface semi-elliptical crack in thick-walled cylinder,” Eng. Fract. Mech., vol. 58, no. 3, pp. 207–221, Oct. 1997, doi: 10.1016/S0013-7944(97)00083-0.

C. P. Andrasic and A. P. Parker, “Dimensionless stress intensity factors for cracked thick cylinders under polynomial crack face loadings,” Eng. Fract. Mech., vol. 19, no. 1, pp. 187–193, Jan. 1984, doi: 10.1016/0013-7944(84)90078-X.

S. R. Mettu, I. S. Raju, and R. G. Forman, “Stress Intensity Factors for Part-Through Surface Cracks in Hollow Cylinders.” 1992.

V. Vullo, “Circular Cylinders and Pressure Vessels,” vol. 3, 2014, doi: 10.1007/978-3-319-00690-1.